Get access to the detailed solutions to the previous years questions asked in IIM IPMAT exam
1 IPMAT 2024 - Quantitative Aptitude
The number of factors of 1800 that are multiple of 6 is ________
2 IPMAT 2024 - Quantitative Aptitude
The number of real solutions of the equation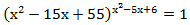 
is _________
3 IPMAT 2024 - Quantitative Aptitude
In a group of 150 students, 52 like tea, 48 like juice and 62 like coffee. If each student in the group likes at least one among tea, juice and coffee, then the maximum number of students that like more than one drink is _____
4 IPMAT 2024 - Quantitative Aptitude
Let ABC be a triangle right-angled at B with AB = BC = 18. The area of largest rectangle that can be inscribed in this triangle and has B as one of the vertices is ______
5 IPMAT 2024 - Quantitative Aptitude
A fruit seller has oranges, apples and bananas in the ratio 3 : 6 : 7. If the number of oranges is a multiple of both 5 and 6, then the minimum number of fruits the seller has is _____
6 IPMAT 2024 - Quantitative Aptitude
The number of pairs (x, y) of integers satisfying the inequality |x − 5|+|y − 5| ≤ 6 is _____
7 IPMAT 2024 - Quantitative Aptitude
The price of a chocolate is increased by x% and then reduced by x%. The new price is 96.76% of the original price. Then x is _____This is an integer type question
8 IPMAT 2024 - Quantitative Aptitude
Let f and g be two functions defined by f(x) = |x + |x|| and g(x) = 1/x for x ≠ 0. If f(a) + g(f(a)) = 13/6 for some real a, then the maximum possible value of f(g(a)) is ______
10 IPMAT 2024 - Quantitative Aptitude
Person A borrows Rs 4000 from another person B for a duration of 4 years. He borrows a portion of it at 3% simple interest per annum, while the rest at 4% simple interest per annum. If B gets Rs 520 as total interest, then the amount A borrowed at 3% per annum in Rs is _______
11 IPMAT 2024 - Quantitative Aptitude
The number of triangles with integer sides and with perimeter 15 is _______
12 IPMAT 2024 - Quantitative Aptitude
If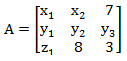 
is a matrix such that the sum of all three elements along any row, column or diagonal are equal to each other, then the value of determinant of A is ______
13 IPMAT 2024 - Quantitative Aptitude
Directions for Qs. 13 - 15: Study the following table and answer the questions based on it
The following table shows the number of employees and their median age in eight companies located in a district.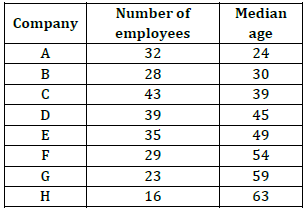
It is known that the age of all employees are integers. It is known that the age of every employee in A is strictly less than the age of every employee in B, the age of every employee in B is strictly less than the age of every employee in C..... the age of every employee in G is strictly less than the age of every employee in H.
The highest possible age of an employee of company A is ______
14 IPMAT 2024 - Quantitative Aptitude
Directions for Qs. 13 - 15: Study the following table and answer the questions based on it
The following table shows the number of employees and their median age in eight companies located in a district.
It is known that the age of all employees are integers. It is known that the age of every employee in A is strictly less than the age of every employee in B, the age of every employee in B is strictly less than the age of every employee in C..... the age of every employee in G is strictly less than the age of every employee in H.
The median age of an employee across the eight companies is ______
15 IPMAT 2024 - Quantitative Aptitude
Directions for Qs. 13 - 15: Study the following table and answer the questions based on it
The following table shows the number of employees and their median age in eight companies located in a district.
It is known that the age of all employees are integers. It is known that the age of every employee in A is strictly less than the age of every employee in B, the age of every employee in B is strictly less than the age of every employee in C..... the age of every employee in G is strictly less than the age of every employee in H.
In company F, the lowest possible sum of the ages of all employees is ______
16 IPMAT 2024 - Quantitative Aptitude
The angle of elevation of the top of a pole from a point A on the ground is 30°. The angle of elevation changes to 45°, after moving 20 metres towards the base of the pole. Then the height of the pole, in metres, is
30
15(√5 + 1)
20(√3 + 1)
10(√3 + 1)
17 IPMAT 2024 - Quantitative Aptitude
If |x + 1| + (y + 2)2 = 0 and ax − 3ay = 1, then the value of a is
1/5
1/2
1/7
2
18 IPMAT 2024 - Quantitative Aptitude
If log4x = a and log25x = b then logx10 is
[a+b]/[2(a−b)]
[a+b]/2
[a+b]/2ab
[a−b]/2ab
19 IPMAT 2024 - Quantitative Aptitude
Let ABC be an equilateral triangle, with each side of length k. If a circle is drawn with diameter AB, then the area of the portion of the triangle lying inside the circle is
(3√3 + π) (k2/6)
(3√3 − π) (k2/24)
(3√3 + π) (k2/24)
(3√3 − π) (k2/6)
20 IPMAT 2024 - Quantitative Aptitude
Let ABC be a triangle with AB = AC and D be a point on BC such that ∠BAD = 30°. If E is a point on AC such that AD= AE, then ∠CDE equals
15°
60°
30°
10°
21 IPMAT 2024 - Quantitative Aptitude
If 5 boys and 3 girls randomly sit around a circular table, the probability that there will be at least one boy sitting between any two girls, is
2/7
1/7
1/4
1/3
22 IPMAT 2024 - Quantitative Aptitude
The side AB of a triangle ABC is c. The median BD is of length k. If ∠BDA = θ < 90°, then the area of triangle ABC is
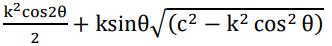
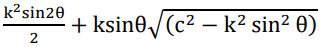
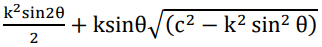
23 IPMAT 2024 - Quantitative Aptitude
Let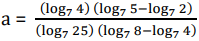 
. Then the value of 5a is
5
8
7/2
5/2
24 IPMAT 2024 - Quantitative Aptitude
For some non-zero real values of a, b and c, it is given that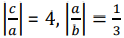 
and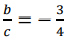 
. If ac > 0, then 
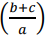equals
7
− 7
− 1
1
25 IPMAT 2024 - Quantitative Aptitude
The difference between the maximum real root and the minimum real root of the equation (x2 − 5)4 + (x2 − 7)4 = 16 is
2√5
2√7
√7
√10
26 IPMAT 2024 - Quantitative Aptitude
If θ is the angle between the pair of tangents drawn from the point A (0, 7/2) to the circle x2 + y2 − 14x + 16y + 88 = 0, then tan θ equals
20/21
5/4
4/5
2/5
27 IPMAT 2024 - Quantitative Aptitude
The numbers 22024 and 52024 are expanded and their digits are written out consecutively on one page. The total number of digits written on the page is
1987
2065
2000
2025
28 IPMAT 2024 - Quantitative Aptitude
A boat goes 96 km upstream in 8 hours and covers the same distance moving downstream in 6 hours. On the next day boat starts from point A, goes downstream for 1 hour, then upstream for 1 hour and repeats this four more time that is, 5 upstream and 5 downstream journeys. Then the boat would be
22.5 km downstream of A
15 km downstream of A
12.5 km downstream of A
20 km downstream of A
29 IPMAT 2024 - Quantitative Aptitude
If the shortest distance of a given point to a given circle is 4 cm and the longest distance is 9 cm, then the radius of the circle is
2.5 cm or 6.5 cm
6.5 cm
5 cm or 13 cm
2.5 cm
30 IPMAT 2024 - Quantitative Aptitude
In a survey of 500 people, it was found that 250 owned a 4-wheeler but not a 2-wheeler, 100 owned a 2-wheeler but not a 4-wheeler, and 100 owned neither a 4-wheeler nor a 2-wheeler. Then the number of people who owned both is
75
60
50
100
31 IPMAT 2024 - Quantitative Aptitude
The sum of a given infinite geometric progression is 80 and the sum of its first two terms is 35. Then the value of n for which the sum of its first n terms is closest to 100, is
4
5
6
7
32 IPMAT 2024 - Quantitative Aptitude
Let n be the number of ways in which 20 identical balloons can be distributed among 5 girls and 3 boys such that everyone gets at least one balloon and no girl gets fewer balloons than a boy does. Then
8000 ≤ n < 9000
7000 ≤ n < 8000
9000 ≤ n < 10000
None of these
33 IPMAT 2024 - Quantitative Aptitude
The greatest number among 2300, 3200, 4100, 2100 + 3100 is
3200
2100 + 3100
4100
2300
34 IPMAT 2024 - Quantitative Aptitude
The number of values of x for which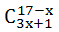 
is defined as an integer is
5
6
2
4
35 IPMAT 2024 - Quantitative Aptitude
The number of solutions of the equation x1 + x2 + x3 + x4 = 50, where x1, x2, x3, x4 are integers with x1 ≥ 1, x2 ≥ 2, x3 ≥ 0, x4 ≥ 0 is
19600
19200
20200
18400
36 IPMAT 2024 - Quantitative Aptitude
Sagarika divides her savings of 10000 rupees to invest across two schemes A and B. Scheme A offers an interest rate of 10% per annum, compounded halfyearly, while scheme B offers a simple interest rate of 12% per annum. If at the end of first year, the value of her investment in scheme B exceeds the value of her investment in scheme A by 2310 rupees, then the total interest, in rupees, earned by Sagarika during the first year of investment is
1100
1130
1111
1000
37 IPMAT 2024 - Quantitative Aptitude
A fruit seller had a certain number of apples, bananas and oranges at the start of the day. The number of bananas was 10 more than the number of apples, and the total number of bananas and apples was a multiple of 11. She was able to sell 70% of apples, 60% of bananas, and 50% of oranges during the day. If she was able to sell 55% of the fruits she had at the start of the day, then the minimum number of oranges she had at the start of the day was
220
190
210
180
38 IPMAT 2024 - Quantitative Aptitude
The terms of a geometric progression are real and positive. If the pth term of the progression is q and the qth term is p, then the logarithm of the first term is
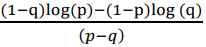
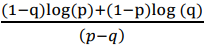
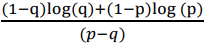
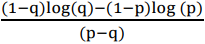
39 IPMAT 2024 - Quantitative Aptitude
The number of real solutions of the equation x2 − 10|x| − 56 = 0 is
4
3
2
1
40 IPMAT 2024 - Quantitative Aptitude
The smallest possible number of students in a class if the girls in the class are less than 50% but more than 48% is
25
100
27
200
41 IPMAT 2024 - Quantitative Aptitude
Directions for Qs. 41 - 45: Read the information and answer the following questions
In an election there were five constituencies S1, S2, S3, S4 and S5 with 20 voters each all of whom voted. Three parties A, B and C contested the elections.
The party that gets maximum number of votes in a constituency wins that seat. In every constituency there was a clear winner. The following additional information is available:
• Total number of votes obtained by A, B and C across all constituencies are 49, 35 and 16 respectively.
• S2 and S3 were won by C while A won only S1.
• Number of votes obtained by B in S1, S2, S3, S4 and S5 are distinct natural numbers in increasing order.
The constituency in which B got lower number of votes compared to A and C is
S4
S1
S3
S2
42 IPMAT 2024 - Quantitative Aptitude
Directions for Qs. 41 - 45: Read the information and answer the following questions
In an election there were five constituencies S1, S2, S3, S4 and S5 with 20 voters each all of whom voted. Three parties A, B and C contested the elections.
The party that gets maximum number of votes in a constituency wins that seat. In every constituency there was a clear winner. The following additional information is available:
• Total number of votes obtained by A, B and C across all constituencies are 49, 35 and 16 respectively.
• S2 and S3 were won by C while A won only S1.
• Number of votes obtained by B in S1, S2, S3, S4 and S5 are distinct natural numbers in increasing order.
The number of votes obtained by B in S2 is
4
5
6
7
43 IPMAT 2024 - Quantitative Aptitude
Directions for Qs. 41 - 45: Read the information and answer the following questions
In an election there were five constituencies S1, S2, S3, S4 and S5 with 20 voters each all of whom voted. Three parties A, B and C contested the elections.
The party that gets maximum number of votes in a constituency wins that seat. In every constituency there was a clear winner. The following additional information is available:
• Total number of votes obtained by A, B and C across all constituencies are 49, 35 and 16 respectively.
• S2 and S3 were won by C while A won only S1.
• Number of votes obtained by B in S1, S2, S3, S4 and S5 are distinct natural numbers in increasing order.
Assume that A and C had formed an alliance and any voter who voted for either A or C would have voted for this alliance. Then the number of seats this alliance would have won is
3
4
5
2
44 IPMAT 2024 - Quantitative Aptitude
Directions for Qs. 41 - 45: Read the information and answer the following questions
In an election there were five constituencies S1, S2, S3, S4 and S5 with 20 voters each all of whom voted. Three parties A, B and C contested the elections.
The party that gets maximum number of votes in a constituency wins that seat. In every constituency there was a clear winner. The following additional information is available:
• Total number of votes obtained by A, B and C across all constituencies are 49, 35 and 16 respectively.
• S2 and S3 were won by C while A won only S1.
• Number of votes obtained by B in S1, S2, S3, S4 and S5 are distinct natural numbers in increasing order.
The number of votes obtained by A in S5 is
7
9
6
8
45 IPMAT 2024 - Quantitative Aptitude
Directions for Qs. 41 - 45: Read the information and answer the following questions
In an election there were five constituencies S1, S2, S3, S4 and S5 with 20 voters each all of whom voted. Three parties A, B and C contested the elections.
The party that gets maximum number of votes in a constituency wins that seat. In every constituency there was a clear winner. The following additional information is available:
• Total number of votes obtained by A, B and C across all constituencies are 49, 35 and 16 respectively.
• S2 and S3 were won by C while A won only S1.
• Number of votes obtained by B in S1, S2, S3, S4 and S5 are distinct natural numbers in increasing order.
Comparing the number votes obtained by A across different constituencies, the lowest number of votes were in constituency
S2
S5
S3
S4